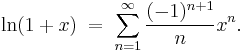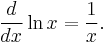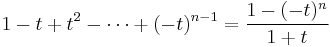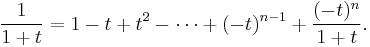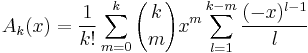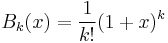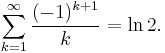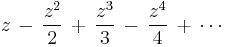Mercator series
In mathematics, the Mercator series or Newton–Mercator series is the Taylor series for the natural logarithm:
The series converges to the natural logarithm (shifted by 1) whenever −1 < x ≤ 1.
Contents |
History
The series was discovered independently by Nicholas Mercator, Isaac Newton and Gregory Saint-Vincent. It was first published by Mercator, in his 1668 treatise Logarithmo-technica.
Derivation
The series can be obtained from Taylor's theorem, by inductively computing the nth derivative of ln x at x = 1, starting with
Alternatively, one can start with the finite geometric series (t ≠ −1)
which gives
It follows that
and by termwise integration,
If −1 < x ≤ 1, the remainder term tends to 0 as 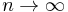 .
.
This expression may be integrated iteratively k more times to yield
where
and
are polynomials in x.[1]
Special cases
Setting x = 1 in the Mercator series yields the alternating harmonic series
Complex series
The complex power series
is the Taylor series for ln(1 + z), where ln denotes the principal branch of the complex logarithm. This series converges within the open unit disk |z| < 1 and on the circle |z| = 1 except at z = -1 (due to Abel's test), and the convergence is uniform on each closed disk of radius strictly less than 1.
References
- Weisstein, Eric W., "Mercator Series" from MathWorld.
- Eriksson, Larsson & Wahde. Matematisk analys med tillämpningar, part 3. Gothenburg 2002. p. 10.
- Some Contemporaries of Descartes, Fermat, Pascal and Huygens from A Short Account of the History of Mathematics (4th edition, 1908) by W. W. Rouse Ball